Odds ratios work the same An odds ratio of 108 will give you an 8% increase in the odds at any value of X Likewise, the difference in the probability (or the odds) depends on the value of X So if you do decide to report the increase in probability at different values of X, you'll have to do it at low, medium, and high values of X Odds ratio in this example is found to be hight () (P The difference between cats and dogs is 3 percentage points or 3 49 ≈ 612 % increase in probability for dogs over cats Meanwhile the odds ratio is 052 1 − 052 049 1 − 049 ≈ 1128 However I am finding a lot of social science literature intpreting O R − 1 as equivalent to increase in probability Example
Statistc 111 Lecture Notes Spring 19 Lecture 11 Odds Ratio Relative Risk Ert2
Odds vs odds ratio vs probability
Odds vs odds ratio vs probability-StATS Odds ratio versus relative risk (created by ) Dear Professor Mean There is some confusion about the use of the odds ratio versus the relative risk Can you explain the difference between these two numbers? Hazard ratio vs odds ratio interpretationAn odds ratio greater than 1 indicates that the condition or event is more likely to occur in the first group And an odds ratio less than 1 indicates that the condition or event is less likely to occur in the first group The odds ratio must be nonnegative if it is defined It is undefined if p 2 q 1




Adjusted Odds Ratios For Age And Sex 95 Confidence Intervals Of Download Table
On the other hand, a gambler backing Manchester United, who have odds of 1/5, will see a payout of just $1 for every $5 bet That means a total payout of $6 for someone betting $5 Bournemouth 5/1 Manchester United 1/5 You Bet $ on Bournemouth You Win $100 ( x 5=100) You also get your $ bet backThe odds aren't as odd as you might think, and the log of the odds is even simpler!RELATIVE RISK AND ODDS RATIO An RR (or OR) more than 10 indicates an increase in risk (or odds) among the exposed compared to the unexposed, whereas a RR (or OR)
e x p ( β) = odds ratio = p 1 1 − p 1 p 2 1 − p 2 I guess what's not coming across is how β, not being a ratio of odds, converts to the odds ratio metric, when taken out of logarithmic space To provide a bit more, if this is the logistic regression equation for the constant l o g ( p 1 − p) = β β 1 ∗ 0 β 2 ∗ 0 β 3 ∗ 0When the disease is rare, the odds ratio will be a very good approximation of the relative risk The more common the disease, the larger is the gap between odds ratio and relative risk In our example above, p wine and p no_wine were 0009 and 0012 respectively, so the odds ratio was a good approximation of the relative risk OR = 0752 and RR If the odds in favor of an event is known, the probability is just the odds divided by one plus the odds ie Probability= Odds/ (1Odds) What is the difference between Probability and Odds?
The Rcode above demonstrates that the exponetiated beta coefficient of a logistic regression is the same as the odds ratio and thus can be interpreted as the change of the odds ratio when we increase the predictor variable \(x\) by one unit In this example the odds ratio"Odds" and "Risk" are the most common terms which are used as measures of association between variables In this article, which is the fourth in the series of common pitfalls in statistical analysis, we explain the meaning of risk and odds and the difference between the twoIn health care it is the ratio of the number of people with the event to the number without It is commonly expressed as a ratio of two integers For example, an odds of 001 is often written as 1100, odds of 033 as 13, and odds of 3 as 31



Epidemiology Stepwards



Plos One Biomarkers Of Host Response Predict Primary End Point Radiological Pneumonia In Tanzanian Children With Clinical Pneumonia A Prospective Cohort Study
It is called that because it is the ratio of two odds Some people call the odds the odds ratio because the odds itself is a ratio That is fine English, but this can quickly lead to confusion If you did that, you would have to call this calculation the odds ratio ratio or the ratio of the odds ratios The odds ratio is the ratio of two odds ODDS RATIO Odds Ratio = Odds of Event A / Odds of Event B For example, we could calculate the odds ratio between picking a red ball and a green ball The probability of picking a red ball is 4/5 = 08 The odds of picking a red ball are (08) / 1(08) = 08 / 02 = 4 The odds ratio for picking a red This is called the odds ratio;



Forest Plot Showing The Pooled Odds Ratio Or Or Hazard Ratio Hr Of Download Scientific Diagram




Logit Wikipedia
Risk ratios, odds ratios, and hazard ratios are three ubiquitous statistical measures in clinical research, yet are often misused or misunderstood in their interpretation of a study's results A 01 paper looking at the use of odds ratios in obstetrics and gynecology research reported 26% of studies (N = 151) misinterpreted odds ratios as risk ratios , while a 12 paper INTRODUCTION Odds ratio (OR) and risk ratio (RR) are two commonly used measures of association reported in research studies In crosssectional studies, the odds ratio is also referred to as the prevalence odds ratio (POR) when prevalent cases are included, and, instead of the RR, the prevalence ratio (PR) is calculatedOdds ratio vs relative risk Odds ratios and relative risks are interpreted in much the same way and if and are much less than and then the odds ratio will be almost the same as the relative risk In some sense the relative risk is a more intuitive measure of effect size Note that the choice is only for prospective studies were the distinctionThe hazard ratio




Odds Vs Probabilities Odds Ratio In Spss Exp B Is An Odds Rather Than A Probability Odds Success Failure Probability Likelihood Of Success For Ppt Download




Forest Plot Of Odds Ratio For The Association Of Family History Of Dm And Hdp In Ethiopia
Smoking The adjusted odds ratio for smoking is calculated as e485 = 1624 This means the odds of having a baby with low birthweight are increased by 624% if the mother smokes (compared to not smoking), assuming the variable age is held constant For example, suppose mother A and mother B are both 30 years oldFractional odds are the ratio of the amount (profit) won to the stake; An Odds Ratio (OR) then is simply the comparison of two odds, OR=Odds (A)/Odds (B) The Relative Risk (RR) is simply the comparison of two risks or probabilities, RR=Probability (A)/Probability (B) This is made more clear when the term is referred to as the Risk Ratio Let's look at this graphically
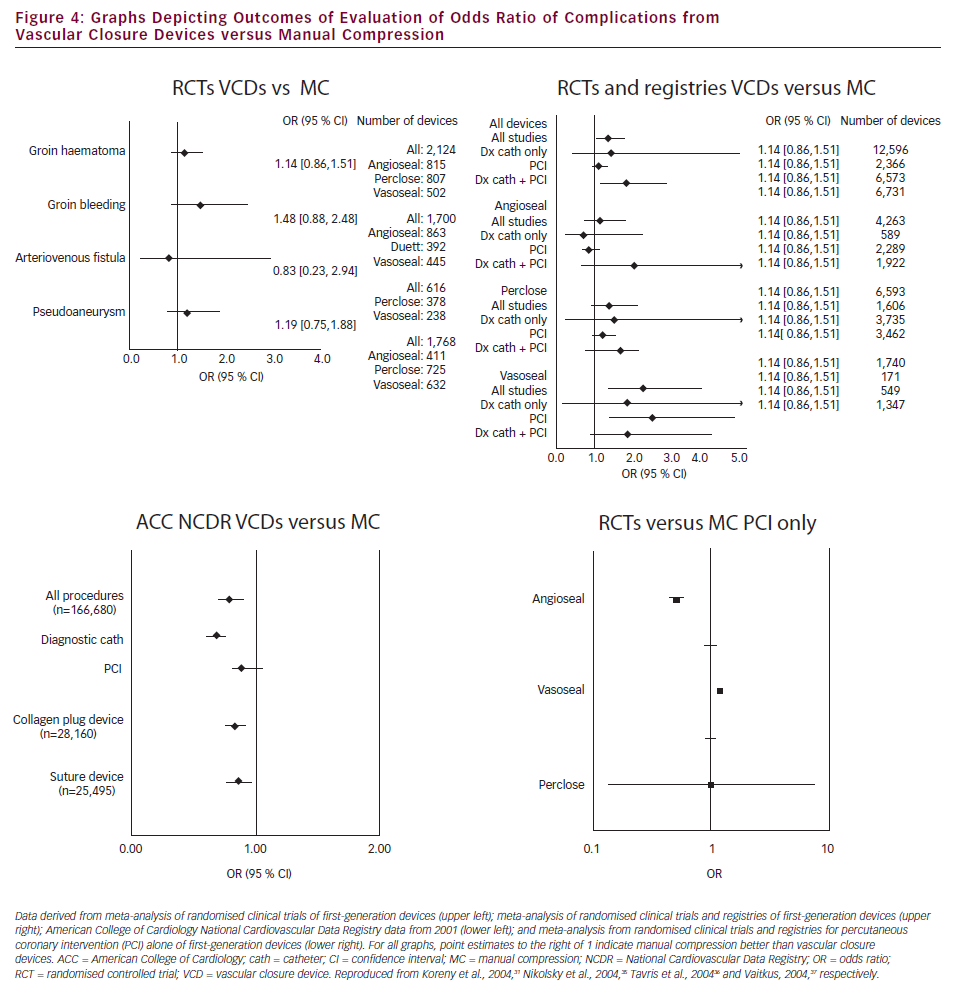



Graphs Depicting Outcomes Of Evaluation Of Odds Ratio Of Complications From Vascular Closure Devices Versus Manual Compression Ecr Journal



Www Jstor Org Stable
Odds Ratios and Log(Odds Ratios) are like RSquared they describe a relationship between two things And just like RSquared, you need to determine if this When the Odds ratio is above 1 and below 2, the likelihood of having the event is represented as XX % higher odds (where XX % is Odds ratio 1) That means that if odds ratio is 124, the likelihood of having the outcome is 24% higher (124 – 1 = 024 ie 24%) than the comparison groupThe odds ratio is defined as the ratio of the odds of an event or disease occurring in one group to the odds occurring in another group The standard formula is X / ( 1 − X) / Y / ( 1 − Y), where X and Y are the probability of that event in the two groups, respectively In contrast, the relative risk is the risk of an event or disease




Ppt Odds Ratio Vs Relative Risk Powerpoint Presentation Free Download Id
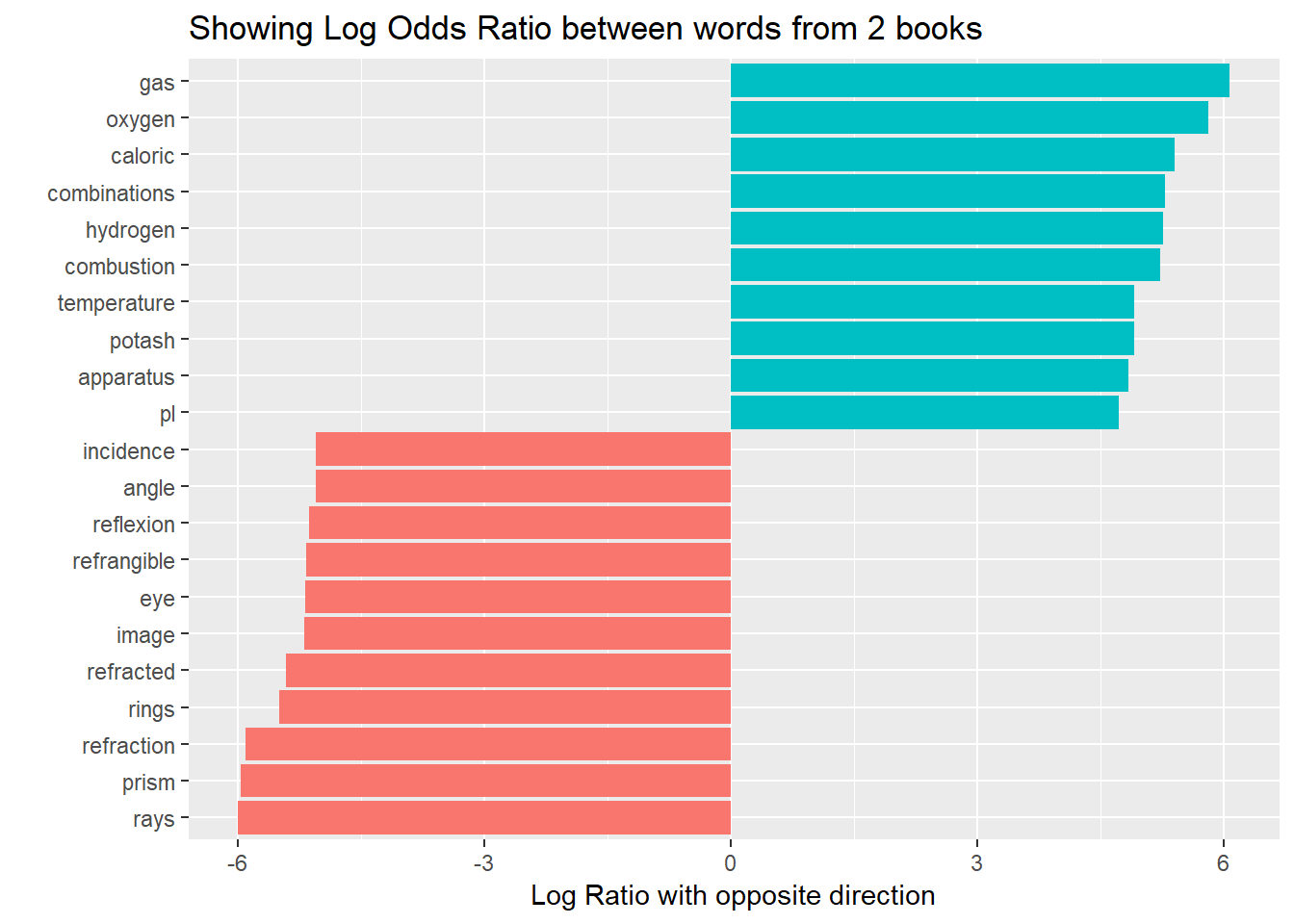



Log Odds Ratio Vs Tf Idf Vs Weighted Log Odds



0 件のコメント:
コメントを投稿