My Partial Derivatives course https//wwwkristakingmathcom/partialderivativescourseIn this video we're talking about how to sketch the level curves ofProblems Elliptic Paraboloid 1 Compute the gradient of w = x 2 5y 2 Answer ∂w ∂w Vw = , = (2x, 10y) ∂x ∂y 2 Show that Vw is perpendicular to the level curves of w at the points (x 0, 0) Answer At (x 0, 0), Vw = (2x 0, 0) Figure 1 The level curves of w = x 2 5y 2 In general, the level curves of w have equation x 2 5y 2We will sketch level curves corresponding to a couples values, such as $0, 1, 1$ The $z=0$ level set is given by $y^2 x= 0$, or $x = y^2$ This is a parabola in $x$ as a function of $y$

Applet Level Curves Of A Hyperbolic Paraboloid Math Insight
Level curves of paraboloid
Level curves of paraboloid-Which of the following graphs depicts the level curves of $z(x,y)=x^2 y$ with values $k=4$ and $k=10$?2 Answers2 Active Oldest Votes 1 In your first example, the proper solution is y = ± k − x 2 You left out the plusorminus That is not a small thing there are usually two values of y for each x, and that greatly affects the plotting of the curves I would say that there is no single general method for finding level curves, in a



Level Sets Ximera
Explain Answer View Answer More Answers 0053 ag Alan G Topics No Related Subtopics Calculus Early Transcendentals (17) Chapter 13 Partial Differentiation Section 1 · One of the easiest curves to create using curve stitching is a parabola The straight lines do not actually create the curve, they merely approximate it The parabola is the envelope of the straight lines This mathematical paper proves that the curve formed by the method below is a parabola Once a parabolic section has been created, you can@8, 8Dµ@8, 8D 30 z =ex 22 y2;
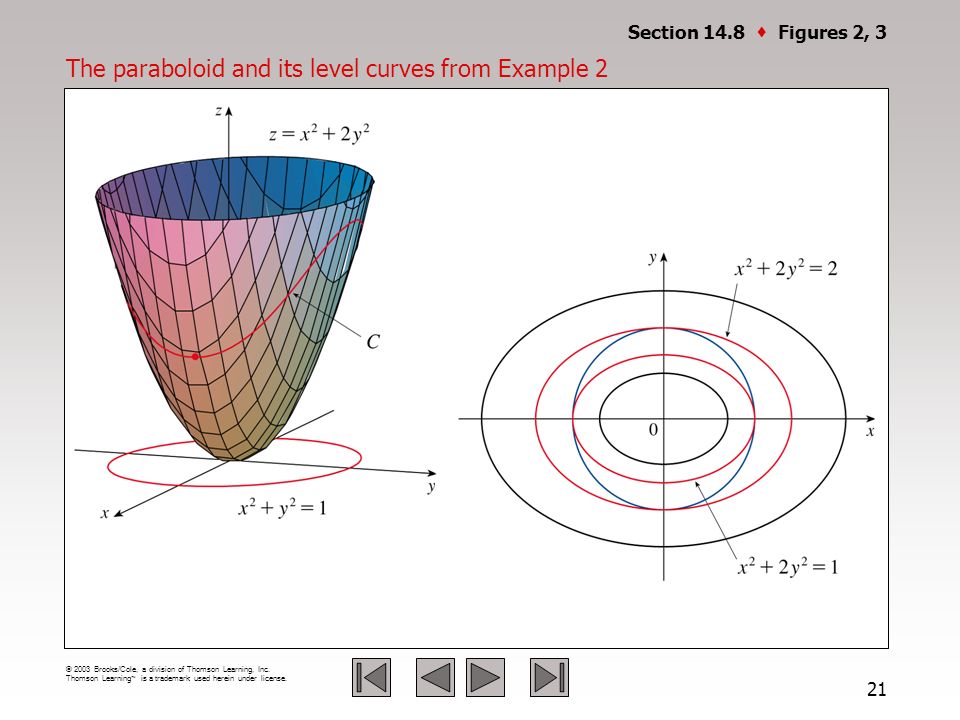
This surface is called a hyperbolic paraboloid because the traces parallel to the x z and y z planes are parabolas and the level curves (traces parallel to the x y plane) are hyperbolas The following figure shows the hyperbolic shape of a level curve To view the interactive graph To rotate the graph, right click and dragThe level curves are circles of the form x2 y2 ° C The level curves are parabolas of the form x2Zo 0 D The level curves are parabolas of the form y2ZoApplet Level curves of an elliptic paraboloid shown with graph Applet loading The graph of the function $f(x,y)=x^22y^2$ is shown is the first panel along with a level curve
Parabola z= x2, matching the graph of the paraboloid 7Explain how to directly con rm that formula card amatches the level curves graph C Solution To nd the level curves, I look at crosssections perpendicular to the zaxis This means setting zto a constant k So all the level curves are of the form k= x2 y2 These areThe other as a contour map in the $xy$plane, the level curves of value $c$ for equally spaced values of $c$ As we shall see, both capture the properties of $z = f(x,\,y)$ from different but illuminating points of view The particular cases of a hyperbolic paraboloid and a paraboloid are shown interactively inThe level curves (in German Niveaukurve, in French ligne de niveau) of a surface z = f(x, y) z = f ( x, y) (1) in R3 ℝ 3 are the intersection curves of the surface and the planes z = constant z = constant Thus the projections of the level curves on the xy x y




Pin On Vector Calculus




Question 1 2 Pts Select All Statements That Are True Chegg Com
Image Elliptic paraboloid level curves Level curves of the elliptic paraboloid $f(x,y)=x^22y^2=c$ for $c=1,2, \ldots, 10$ These curves are ellipses of increasing size Image file elliptic_paraboloid_level_curvespng Image links This image is found in the pages · First, set z = 0, and then graph that equation That will be the level curve for z = 0 Second, set z = 1, and then graph that curve You will have a different equation for each level curve Cottontails said However, what I am unsure of how is to how to actually sketch the level curves and then find at what values would z = 0 and z = 1 be drawnShow that the level curves of the cone {eq}z = (x^2 y^2)^{\dfrac 1 2} {/eq} and the paraboloid {eq}z = x^2 y^2 {/eq} are circles Level Curves To solve this



Chapter 14 Partial Derivatives Ppt Video Online Download



Hyperbolic Paraboloid
Third The gradient vector is orthogonal to level sets In particular, given , the gradient vector is always orthogonal to the level curves Moreover, given , is always orthogonal to level surfaces Computing the gradient vector Given a function of several variables, say , the gradient, when evaluated at a point in the domain of , is a vectorIn mathematics, a parabola is a plane curve which is mirrorsymmetrical and is approximately UshapedIt fits several superficially different mathematical descriptions, which can all be proved to define exactly the same curves One description of a parabola involves a point (the focus) and a line (the directrix)The focus does not lie on the directrix The parabola is the locus of points inLevel curves and contour plots are another way of visualizing functions of two variables If you have seen a topographic map then you have seen a contour plot Example To illustrate this we first draw the graph of z = x2 y2 On this graph we draw contours, which are curves at a fixed height z = constant For example the curve at height z = 1 is the circle x2 y2 = 1 On the graph we have




13 1 Functions Of Several Variables Mathematics Libretexts



13 1
The level curves of this function are ellipses centered at (1, 2) The semimajor axis of each ellipse is vertical and the semiminor axis is horizontal That is, the ellipses are taller than they are wide, and so this option doesn't match the given set of curvesLevel Curves Author Kristen Beck Topic Functions This worksheet illustrates the level curves of a function of two variables You may enter any function which is a polynomial in both andParabolic curve such that its lowest point is directly below "P"with a vertical clearance of 55 m Stationing of the PI is 5 800 and has an elevation of 105 m The slope of the tangent passing thru the PC is 4% and that of the PT is 3% Determine the (a) length of the vertical parabolic curve (b) stationing of point "P"being



Contours Html




The Hyperbolic Paraboloid The Curve Of Weighted Fitness Function F 0 Download Scientific Diagram
@6, 6Dµ@6, 6D 32 z = yx21 ;The other as a contour map in the $xy$plane, the level curves of value $c$ for equally spaced values of $c$ As we shall see, both capture the properties of $z = f(x,\,y)$ from different but illuminating points of view The particular cases of a hyperbolic paraboloid and a paraboloid are shown interactively inThe conic sections, from left to right, are an ellipse, a hyperbola and a parabola Curves Circles The simplest nonlinear curve is unquestionably the circle A circle with center (a,b) and radius r has an equation as follows (x a) 2 (x b) 2 = r 2 If the center is




Functions Of Several Variables




Paraboloid Wikipedia



0 件のコメント:
コメントを投稿